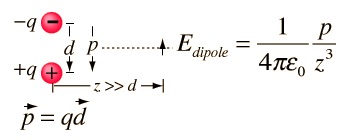
An electric dipole is made up of two point charges that have equal but opposite electric charges (q) and are separated by a short distance (d).
The electric field is inversely proportional to the cube of the distance from the dipole, and is dependent on whether you’re moving along the line separating the two charges or perpendicular to it.
A dipole can be created, for example, when you place a neutral atom in an electric field, because of the movement of electrons, the atom polarizes (negative charge on one side, positive charge on the other) and yields a separation of charge.
Electric dipole can be characterized by its dipole moment, which is a measure of the separation of positive and negative electrical charges within a system. Two point charges, one with charge +q and the other one with charge −q separated by a distance d, constitute an electric dipole. The magnitude of the dipole moment is:
A prime example of this is the molecule for water, which forms a 105 degree angle between the two hydrogens connected to the oxygen. Since the oxygen has a greater electronegativity, it pulls more strongly on the electrons shared by the oxygen and hydrogen atoms and that end of the molecule becomes more negatively charged compared to the hydrogen end. Therefore, the net electric dipole points towards the oxygen atom.

An electric dipole is constructed from two point charges, one at position [math]\displaystyle< [\frac, 0] >[/math] and one at position [math]\displaystyle< [\frac, 0] >[/math] . These point charges are of equal and opposite charge. We then wish to know the electric field due to the dipole at some point [math]\displaystyle< p >[/math] in the plane (see the figure). [math]\displaystyle< p >[/math] can be considered either a distance [math]\displaystyle< [x_0, y_0] >[/math] from the midpoint of the dipole, or a distance [math]\displaystyle< r >[/math] and an angle [math]\displaystyle< \theta >[/math] as in the diagram.
We state that the net electric field at [math]\displaystyle< p >[/math] is [math]\displaystyle < E_>[/math] and has an x and y component, [math]\displaystyle < E_>[/math] and [math]\displaystyle < E_>[/math] . Then we can individually calculate the x and y components. First we realize that since [math]\displaystyle < E_= E_ + E_ >[/math] , [math]\displaystyle < E_= E_> + E_> >[/math] , similarly, for y, [math]\displaystyle < E_= E_> + E_> >[/math] . At this point, it's worth noting that [math]\displaystyle< E_> = E_ * cos(\theta_+) >[/math] , where [math]\displaystyle< \theta_+ >[/math] is the angle from [math]\displaystyle < q_>[/math] to [math]\displaystyle< p >[/math] .
[math]\displaystyle< \theta_+ >[/math] and its counterpart [math]\displaystyle< \theta_- >[/math] are not known. However, we can calculate them. We know [math]\displaystyle< \theta_+ >[/math] is formed by a triangle with one side length [math]\displaystyle< p_y >[/math] and one side length [math]\displaystyle< p_x - \frac >[/math] . Then [math]\displaystyle< sin(\theta_+) = \frac<\sqrt<(p_x - \frac)^2+p_y^2>> >[/math] , from which you can calculate the angle. This looks disgusting, but a close inspection shows that [math]\displaystyle< p_y >[/math] is the opposite side of the triangle, and the denominator is an expression forming the hypotenuse of the triangle ( [math]\displaystyle< r_+ >[/math] ) from known quantities. A similar method shows that [math]\displaystyle< sin(\theta_-) = \frac<\sqrt<(p_x + \frac)^2+p_y^2>> >[/math] , where once again [math]\displaystyle< \sqrt<(p_x + \frac)^2+p_y^2> = |\vec r_-| >[/math] .
We now have values for [math]\displaystyle< d, q, \theta_+, \theta_-, \vec r_+, \vec r_- >[/math] . This is enough to calculate [math]\displaystyle < E_>[/math] in both directions. The general formula for electric field strength from a Point Charge is [math]\displaystyle< E = \frac <4\pi\epsilon_0>\frac <|\vec r|^2>\hat r >[/math] . Then [math]\displaystyle< |E_+| = \frac <4\pi\epsilon_0>\frac <|\vec r_+|^2>>[/math] and [math]\displaystyle< |E_-| = \frac <4\pi\epsilon_0>\frac <|\vec r_-|^2>>[/math] . We want solely the magnitude in this case because we can calculate direction and component forces using sin and cosine. It's worth noting that we can expand [math]\displaystyle< r_+, r_- >[/math] to the form in the denominator of the sine and cosine. We will use this later.
Then we combine some terms, noting that [math]\displaystyle< q_+ = -q_- >[/math] . [math]\displaystyle < E_= \frac <4\pi\epsilon_0>* \Bigg(\frac<|\vec r_+|^2>sin(\theta_+) + \frac<|\vec r_-|^2>sin(\theta_-)\Bigg) >[/math]
Finally we can combine more terms, the denominators of the expanded sines are the square roots of the radii. We can also pull out the negative sign.
Much of the derivation for the x direction is similar. The major difference is that instead of calculating the sine, opposite over hypotenuse, we want cosine, adjacent over hypotenuse. That is, where [math]\displaystyle< sin(\theta_+) = \frac<\sqrt<(p_x - \frac
[math]\displaystyle < E_= \frac <4\pi\epsilon_0>\Bigg( \frac>< \Big((p_x - \frac)^2 +p_y^2 \Big)^\frac > - \frac
We can simplify the solution for many cases
On the parallel axis, we begin with the now known formula [math]\displaystyle < E_= \frac <4\pi\epsilon_0>\Bigg( \frac>< \Big((p_x - \frac)^2 +p_y^2 \Big)^\frac > - \frac
Then, combining exponents and reducing the fraction: [math]\displaystyle < E_= \frac <4\pi\epsilon_0>\Bigg( \frac< (p_x - \frac)^2 > - \frac< (p_x + \frac)^2 > \Bigg) >[/math] .
Then, we can combine these fractions. to simplify the calculations, replace [math]\displaystyle< \frac >[/math] with [math]\displaystyle< a >[/math] .
This is the formula. When [math]\displaystyle< p_x \gt \gt a >[/math] , we can assume that [math]\displaystyle< p_x^2 + a^2 >[/math] is very close to [math]\displaystyle< p_x^2 >[/math] . Then
We can do a similar simplification for the perpendicular axis. We know that [math]\displaystyle < E_= 0 >[/math] because the vertical forces from both point charges cancel, leaving only horizontal forces.
In this case though, [math]\displaystyle< p_x = 0 >[/math]
Once again, we say [math]\displaystyle< a = \frac >[/math] .
And this is our result.
Once again, when [math]\displaystyle< d >[/math] is much smaller than [math]\displaystyle< p_y >[/math] , [math]\displaystyle< a >[/math] is also small, so we can assume that the denominator is just [math]\displaystyle< p_y >[/math] . This allows us to simplify the resulting equation to
A dipole is located at the origin, and is composed of charged particles with charge [math]\displaystyle< +e >[/math] and [math]\displaystyle< -e >[/math] , separated by a distance [math]\displaystyle < 9 \times10^>[/math] along the [math]\displaystyle< y >[/math] axis. The [math]\displaystyle< +e >[/math] charge is on the [math]\displaystyle< +y >[/math] axis. Calculate the force on a proton due to this dipole at a location [math]\displaystyle < \lt 0, 0, 3 \times 10^\gt >[/math] meters.
The center of the dipole is at the origin and there is a proton along the z axis. In this case, we apply the perpendicular from of the electric field equation. In this case, since [math]\displaystyle< r \gt \gt d >[/math] , we can also use an approximate solution. Therefore, we apply the formula [math]\displaystyle < E_= \frac <4\pi\epsilon_0>\frac >[/math] . Since [math]\displaystyle< a = \frac >[/math] , and r is the distance to the proton, we can plug in the values and solve for the net electric field.

[math]\displaystyle < 1.6\times 10^\times 9 \times 10^9 \frac> > = -48000 \frac >[/math] on the y axis, as a vector: [math]\displaystyle< \lt 0, -48000, 0\gt >[/math] .
However, we aren't done since we want to know the force. We know that [math]\displaystyle< F = qE >[/math] and in this case, both [math]\displaystyle< q >[/math] , the charge on the proton and [math]\displaystyle< E >[/math] , the electric field, are known. Thus the solution is [math]\displaystyle < -48000 \times 1.6 \times 10^= -7.68 \times 10^ >[/math] on the y axis, or [math]\displaystyle< \lt 0, -7.68 \times 10^, 0\gt >[/math] .
A ball of mass [math]\displaystyle< M >[/math] and radius [math]\displaystyle< R >[/math] is given an unknown negative charge spread uniformly over its surface. The ball is hanging from a thread and can move freely. A distance [math]\displaystyle< L >[/math] directly below the center of the ball, a small permanent dipole is oriented such that the dipole axis is parallel with the center of the ball. The dipole has a dipole moment [math]\displaystyle< p = qs >[/math] , with a distance [math]\displaystyle< s >[/math] between the positive and negative charges of the dipole, and a mass [math]\displaystyle< m >[/math] . The positive charge of the dipole is oriented closer to the center of the ball.
a) calculate the charge on the ball to levitate the dipole
b) the dipole is turned 90 degrees clockwise, without changing its position relative to the ball, what effect does this have on the ball?
a) Because the dipole is small, we can assume that [math]\displaystyle< s \lt \lt L >[/math] . We wish to find the force on the dipole such that it can equal the force due to gravity. Once again, [math]\displaystyle< F = qE >[/math] since by newton's third law, for a force exerted on the ball by the dipole, there is an equal and opposite for exerted on the dipole by the ball. That is [math]\displaystyle< F_G = F_E >[/math] , so [math]\displaystyle< qE = mg >[/math] (where [math]\displaystyle< g >[/math] is the acceleration due to gravity). Therefore, in this case we wish to find the force on the ball, meaning the electric field from the dipole and the charge on the ball, [math]\displaystyle< Q >[/math] . The field from the dipole is, since we are on the parallel axis, [math]\displaystyle< E = \frac <4\pi\epsilon_0>\frac >[/math] . Putting this together, we get [math]\displaystyle< mg = |Q| \frac <4\pi\epsilon_0>\frac >[/math]
However, we know that since the positive charge of the dipole is closer to the ball, the charge on the ball must be negative to create an attractive force. [math]\displaystyle< |Q| \gt 0 >[/math] , so our final answer is [math]\displaystyle< Q = -\Bigg(\frac<4\pi\epsilon_0>\Bigg)^ \frac >[/math]
b) By rotating the dipole clockwise the direction of the electric field at the location of the ball changes. Since the positive end of the dipole is to the right, and the negative end to the left of the dipole, the electric field from the dipole acting on the ball is oriented toward the left. However, since the ball has negative charge, this results in a force on the ball to the right.
Is it possible for a permanent electric dipole to have a net (total) charge of zero?
Permanent dipoles occur when two atoms in a molecule have a great difference in their electronegativity; one atom attracts electrons more than the other, becoming more negative, while the other atom becomes more positive. A permanent magnet, such as a bar magnet, owes its magnetism to the magnetic dipole moment of the electron. A molecule with a permanent dipole moment is called a polar molecule. A molecule is polarized when it carries an induced dipole. A non-degenerate (S-state) atom can have only a zero permanent dipole.
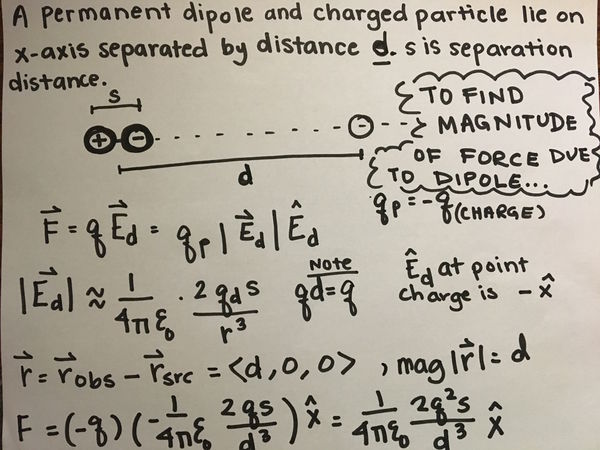
The electric field of an electric dipole can be constructed as a vector sum of the point charge fields of the two charges. As can be seen in the graphics, the electric field always points towards the negative particle and points away from the positive particle. This is an important characteristic which can be used to determine which end is positive and which is negative in a dipole.
Direction of electric dipole:
In this class, most of the questions with either be on axis or perpendicular to the dipole, so learning the direction of the electric field at these locations is important. This is done by decomposing the two vectors from each charged particle of the dipole, as shown in the positive x-axis location in the picture below. Where the positive particle has an electric field pointing away, and the negative particle electric field points inward.

Consider a dipole with the following arbitrary orientation in a uniform electric field:
Note how the electric field will exert an an electric force on each of the point charges. Since the electric field is uniform and charge of each point charge is equal and opposite, the electric force exerted on each point charge will be equal and opposite. The magnitude of each force is simply the force on a point charge, or [math]\displaystyle< F = qE >[/math] . The component of this force perpendicular to the dipole axis can be written as [math]\displaystyle< F_= qE\sin \theta >[/math] , where theta is the angle between the electric field and the dipole. It is this perpendicular force which causes rotational motion, and thus is the force component of the applied torque. Since the forces are separated by the distance of the dipole, it can be generalized that an electric field produces the following torque on an electric dipole:
The direction of the torque can be found using the right hand rule, as it will always be perpendicular to the dipole axis and applied force. The cross product relationship also indicates that when the dipole is parallel to the electric field, no torque will be acting on it.
It is important to note that since the forces on each of the point charges are equal and opposite, the net force on the dipole in a uniform field is 0, which also explains why there is no linear motion by the dipole. Also, the torque from the electric field will align the orientation of the dipole to be parallel with the electric field. This is because at this point the force applied to each of the point charges is 0 because [math]\displaystyle< F = Eqd \sin 0 = 0 >[/math] .
The torque that rotates the dipole moves the orientation of the dipole from a higher energy configuration to a lower energy configuration as shown below.
This also means that any rotation done against this energy gradient requires work. The amount of work required to rotate a dipole from its low energy configuration to an angle [math]\displaystyle< \theta_0 >[/math] can be derived as:
[math]\displaystyle< \int_^\tau d\theta = \int_^Eqd\sin\theta d\theta = \int_^Ep\sin\theta d\theta = Ep(1-\cos\theta_0) >[/math]
Calculating potential energy is a matter of convention. The standard convention it the potential energy is zero when the dipole is perpendicular to the electric field. With this in mind, the potential energy of a dipole can be calculated as:
Again, this would indicate the potential energy is maximized when [math]\displaystyle< \cos\theta = 1 >[/math] , or when the dipole is in the low energy configuration.
When the electric field is not uniform, the two point charges will not feel equal and opposite forces at all times, meaning that the net force on the dipole will not be zero. The force on the dipole will be in the direction of where the electric field has the steepest increase. The derivation for the net force on a dipole in a non-uniform electric field is extremely complex [1], but can be explained in simple terms. Consider an non-uniform electric field E with a gradient [math]\displaystyle< \nabla >[/math] . For any dipole placed in this system, the difference between the electric field at small intervals between the two point charges can be expanded as a power series to calculate the total difference in electric field. It can be proven that in an ideal dipole, all the subleading terms vanish, and all that is left is the leading term of this series. Thus, the general formula for net force on a dipole in a non-uniform electric field can be written as:
where [math]\displaystyle< E(r) >[/math] is the electric field at the center of the dipole.
This concept map illustrates the other fields and forces caused by the electric dipole.
Dipoles are incredibly common in physics, chemistry, and other natural sciences. While not specific to electric dipoles, much of the mathematics taught in advanced algorithms is relevant to the study of dipoles in nature, specifically certain randomized algorithms useful in computer science can be used to effectively simulate and predict natural phenomena having to do with dipole forces and the arrangement of many dipoles.
Dipoles are useful in determining the behavior of certain molecules with each other. Polar molecules can act as electric dipoles, such as the water molecule mentioned earlier. This gives polar molecules certain properties when in a solution. Dipoles are the basis for polarity in molecules, which leads to other important properties such as hydrophilicity, which is very important in industry as well as in your body. The cells in the body are surrounded by a selectively permeable membrane. The outer and inner ends of this membrane are polar, while the middle part is non polar. This polarity is very important in determining which molecules enter and exit the cells in our body and therefore how the cells maintain homeostasis. Dipoles are also very common in regards to magnets, which have several applications including Maglev trains and even metal detectors.
To specifically Biomedical Engineering, dipole interactions can help break down molecules as a result of ion polarization and separation.
Electric dipoles have been understood since the mid to late 1800s. However, atomic dipoles could only be understood after the discovery of the correct model of the atom by Bohr in 1913. Based on this knowledge, atomic dipoles were used in a lot of technology. Even though electric dipoles are a newer concept, the human understanding of magnetic dipoles goes way back to the ancient Greeks who discovered magnetite, which had magnetic properties.